310363: CF1822C. Bun Lover

C. Bun Lovertime limit per test3 secondsmemory limit per test256 megabytesinputstandard inputoutputstandard output

Tema loves cinnabon rolls — buns with cinnabon and chocolate in the shape of a "snail".

Cinnabon rolls come in different sizes and are square when viewed from above. The most delicious part of a roll is the chocolate, which is poured in a thin layer over the cinnabon roll in the form of a spiral and around the bun, as in the following picture:

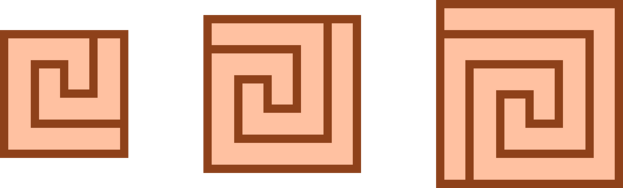
Cinnabon rolls of sizes 4, 5, 6

For a cinnabon roll of size $n$, the length of the outer side of the square is $n$, and the length of the shortest vertical chocolate segment in the central part is one.

Formally, the bun consists of two dough spirals separated by chocolate. A cinnabon roll of size $n + 1$ is obtained from a cinnabon roll of size $n$ by wrapping each of the dough spirals around the cinnabon roll for another layer.

It is important that a cinnabon roll of size $n$ is defined in a unique way.

Tema is interested in how much chocolate is in his cinnabon roll of size $n$. Since Tema has long stopped buying small cinnabon rolls, it is guaranteed that $n \ge 4$.

Answer this non-obvious question by calculating the total length of the chocolate layer.

Input

The first line of the input contains a single integer $t$ ($1 \le t \le 10^5$) — the number of test cases.

The following $t$ lines describe the test cases.

Each test case is described by a single integer $n$ ($4 \le n \le 10^9$) — the size of the cinnabon roll.

Output

Output $t$ integers. The $i$-th of them should be equal to the total length of the chocolate layer in the $i$-th test case.

ExampleInput

4
4
5
6
179179179

Output

26
37
50
32105178545472401

题目大意：
Tema非常喜欢肉桂卷——一种螺旋形状，内含肉桂和巧克力的面包。肉桂卷有不同的尺寸，从顶部看是正方形的。肉桂卷上最可口的部分是巧克力，它以薄层的形式螺旋状地覆盖在肉桂卷上，并围绕面包，如下图所示：

对于尺寸为 $ n $ 的肉桂卷，正方形的外边长为 $ n $，中心部分最短的垂直巧克力段的长度为1。形式上，面包由两个面团的螺旋和它们之间的巧克力层组成。尺寸为 $ n+1 $ 的肉桂卷是由尺寸为 $ n $ 的肉桂卷通过将每个面团的螺旋再围绕肉桂卷包裹一层得到的。

重要的是，尺寸为 $ n $ 的肉桂卷是以唯一的方式定义的。

Tema对尺寸为 $ n $ 的肉桂卷中巧克力的总量感兴趣。由于Tema已经很久不买小尺寸的肉桂卷了，所以保证 $ n \ge 4 $。

通过计算巧克力层的总长度来回答这个不太明显的问题。

输入输出数据格式：
输入：
第一行包含一个整数 $ t $ ($ 1 \le t \le 10^5 $) —— 测试用例的数量。
接下来的 $ t $ 行描述测试用例。
每个测试用例由一个整数 $ n $ ($ 4 \le n \le 10^9 $) 描述 —— 肉桂卷的尺寸。

输出：
输出 $ t $ 个整数。第 $ i $ 个数应等于第 $ i $ 个测试用例中巧克力层的总长度。题目大意： Tema非常喜欢肉桂卷——一种螺旋形状，内含肉桂和巧克力的面包。肉桂卷有不同的尺寸，从顶部看是正方形的。肉桂卷上最可口的部分是巧克力，它以薄层的形式螺旋状地覆盖在肉桂卷上，并围绕面包，如下图所示： 对于尺寸为 $ n $ 的肉桂卷，正方形的外边长为 $ n $，中心部分最短的垂直巧克力段的长度为1。形式上，面包由两个面团的螺旋和它们之间的巧克力层组成。尺寸为 $ n+1 $ 的肉桂卷是由尺寸为 $ n $ 的肉桂卷通过将每个面团的螺旋再围绕肉桂卷包裹一层得到的。 重要的是，尺寸为 $ n $ 的肉桂卷是以唯一的方式定义的。 Tema对尺寸为 $ n $ 的肉桂卷中巧克力的总量感兴趣。由于Tema已经很久不买小尺寸的肉桂卷了，所以保证 $ n \ge 4 $。 通过计算巧克力层的总长度来回答这个不太明显的问题。 输入输出数据格式： 输入： 第一行包含一个整数 $ t $ ($ 1 \le t \le 10^5 $) —— 测试用例的数量。 接下来的 $ t $ 行描述测试用例。 每个测试用例由一个整数 $ n $ ($ 4 \le n \le 10^9 $) 描述 —— 肉桂卷的尺寸。 输出： 输出 $ t $ 个整数。第 $ i $ 个数应等于第 $ i $ 个测试用例中巧克力层的总长度。