Mirror Grid

题意翻译

给定一个 $n\times n\ (n\le100)$ 的 01 矩形，求至少修改多少次后能使矩形旋转 0°，90°，180°，270°后所形成的矩形都完全相同。

题目描述

You are given a square grid with $ n $ rows and $ n $ columns. Each cell contains either $ 0 $ or $ 1 $ . In an operation, you can select a cell of the grid and flip it (from $ 0 \to 1 $ or $ 1 \to 0 $ ). Find the minimum number of operations you need to obtain a square that remains the same when rotated $ 0^{\circ} $ , $ 90^{\circ} $ , $ 180^{\circ} $ and $ 270^{\circ} $ . The picture below shows an example of all rotations of a grid.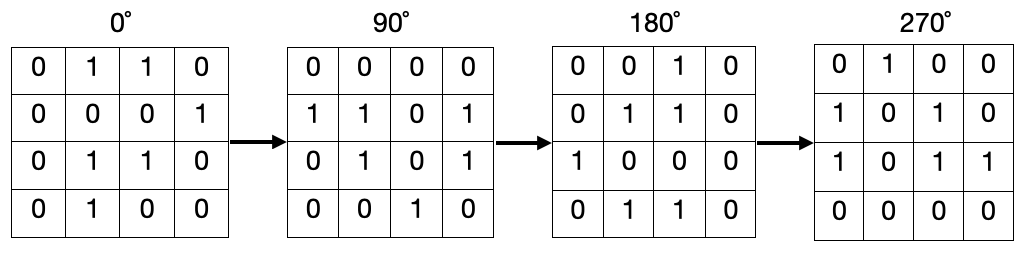 

输入输出格式

输入格式

The first line contains a single integer $ t $ ( $ 1 \leq t \leq 100 $ ) — the number of test cases. The first line of each test case contains a single integer $ n $ ( $ 1 \leq n \leq 100 $ ) — the size of the grid. Then $ n $ lines follow, each with $ n $ characters $ a_{i,j} $ ( $ 0 \leq a_{i,j} \leq 1 $ ) — the number written in each cell.

输出格式

For each test case output a single integer — the minimum number of operations needed to make the square look the same rotated $ 0^{\circ} $ , $ 90^{\circ} $ , $ 180^{\circ} $ and $ 270^{\circ} $ .

输入输出样例

输入样例 #1

5
3
010
110
010
1
0
5
11100
11011
01011
10011
11000
5
01000
10101
01010
00010
01001
5
11001
00000
11111
10110
01111

输出样例 #1

1
0
9
7
6

说明

In the first test case, we can perform one operations to make the grid $ \begin{matrix}0 & 1 & 0\\ 1 & 1 & \color{red}{1}\\ 0 & 1 & 0\end{matrix} $ . Now, all rotations of the square are the same. In the second test case, all rotations of the square are already the same, so we don't need any flips.

题意翻译

给定一个 $n\times n\ (n\le100)$ 的 01 矩形，求至少修改多少次后能使矩形旋转 0°，90°，180°，270°后所形成的矩形都完全相同。