Remainders Game

题意翻译

给定正整数 $n,k$ 和 $n$ 个正整数 $c_1,c_2,\cdots,c_n$。如果对于任意正整数 $x$，可以通过 $x\mod c_i$ 的值推出 $x\mod k$ 的值则输出 `Yes` 否则输出 `No`。

题目描述

Today Pari and Arya are playing a game called Remainders. Pari chooses two positive integer $ x $ and $ k $ , and tells Arya $ k $ but not $ x $ . Arya have to find the value 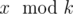. There are $ n $ ancient numbers $ c_{1},c_{2},...,c_{n} $ and Pari has to tell Arya 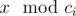 if Arya wants. Given $ k $ and the ancient values, tell us if Arya has a winning strategy independent of value of $ x $ or not. Formally, is it true that Arya can understand the value 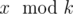 for any positive integer $ x $ ? Note, that 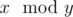 means the remainder of $ x $ after dividing it by $ y $ .

输入输出格式

输入格式

The first line of the input contains two integers $ n $ and $ k $ ( $ 1<=n,\ k<=1000000 $ ) — the number of ancient integers and value $ k $ that is chosen by Pari. The second line contains $ n $ integers $ c_{1},c_{2},...,c_{n} $ ( $ 1<=c_{i}<=1000000 $ ).

输出格式

Print "Yes" (without quotes) if Arya has a winning strategy independent of value of $ x $ , or "No" (without quotes) otherwise.

输入输出样例

输入样例 #1

4 5
2 3 5 12

输出样例 #1

Yes

输入样例 #2

2 7
2 3

输出样例 #2

No

说明

In the first sample, Arya can understand  because $ 5 $ is one of the ancient numbers. In the second sample, Arya can't be sure what  is. For example $ 1 $ and $ 7 $ have the same remainders after dividing by $ 2 $ and $ 3 $ , but they differ in remainders after dividing by $ 7 $ .